Multiple Stereocenters

MULTIPLE STEREOCENTERS

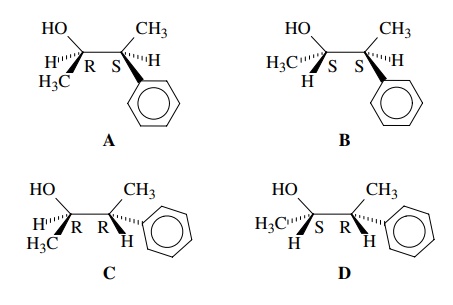
When there is more than one chiral center in a molecule, the number of possible stereoisomers increases. Since each chiral center can have either the R or S configuration, for a molecule of n chiral centers, there will be 2ⁿ possi-ble stereoisomers. Thus 3-phenyl-2-butanol has two stereogenic centers and four possible stereoisomers. These are shown below with the configuration of each chiral center designated.

The configuration of each chiral center can be determined in the usual way, but there is a much faster way to draw the four stereoisomers. First draw a stereostructure of the molecule such as A and then go through the process of determining the R or S configuration. Each chiral center is a stereogenic center in that the interchange of two ligands results in a stereoisomer. Since each chiral center can only be R or S and the configuration of a particular chiral center can be changed merely by exchanging any two valences, the remaining stereoisomers of A can now be generated easily by switching valences of one stereocenter (B), then the other (C), then both (D). Furthermore, if the relative configurations (R,S) are known for A, then the configurations of the other stereoisomers are immediately known since exchanging any two valences inverts the configuration (R goes to S). For 3-phenyl-2-butanol, structure A has the configuration (2R,3S)-3-phenyl-2-butanol. (The stereochemical information is denoted by the number of the carbon and its configuration enclosed in parentheses before the name of the compound.) By exchanging the hydrogen and methyl groups C-2 of A, the configuration of C-2 is inverted and isomer B is produced, the 2S,3S isomer. Exchange of the hydrogen and phenyl groups at C-3 of A inverts the configuration of C-3 and isomer C is produced, the 2R,3R isomer. Exchanging the hydrogen and methyl group at C-2 and the hydrogen and phenyl group at C-3 of A gives isomer D, the 2S,3R isomer.

While the exchange of the methyl and hydrogen groups at C-2 of A was used to invert the configuration, the same configurational change at C-2 of A could be accomplished by exchanging any two valences, such as hydrogen and hydroxyl or hydroxyl and methyl. The same is true for configurational changes at C-3. Exchange of any two ligands will invert the configuration of a tetrahedral stereogenic center.

Thus, given the configurations for the three chiral centers of an aldopentose such as D, one can rapidly write down the configurations of the chiral centers of E, F, or G,

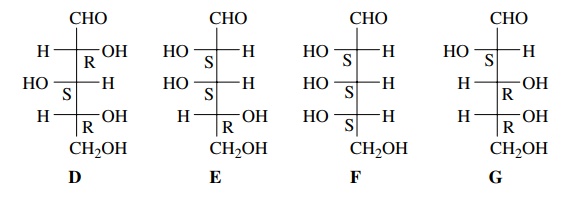
which are stereoisomers of D, without having to apply the Cahn – Ingold – Prelog rules for each isomer. This is done by simply noting which substituents are switched relative to those in D. If the configuration is switched from that in D, then the designation (R,S) will also be switched from that in D. Isomers D–G are only four stereoisomers out of eight possible stereoisomers for an aldopentose which has three stereocenters (2³ = 8).

Now that we can generate the stereoisomers of compounds with more than one chiral center, it is appropriate to ask what are the relationships between these isomers. Thus 2-bromo-3-acetoxy butane has two chiral centers and four stereoisomers, shown below.

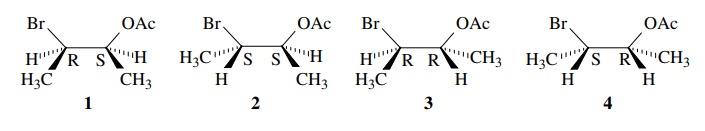
Since each carbon of 1 is the mirror-image configuration of the carbons in 4 (i.e., C-2 of 1 is R and C-2 of 4 is S; C-3 of 1 is S and C-3 of 4 is R), then the molecules themselves are mirror images, but they are nonsuperimposable. They are thus enantiomers. This relationship can also be shown by reorienting the molecules to see that they are mirror images,

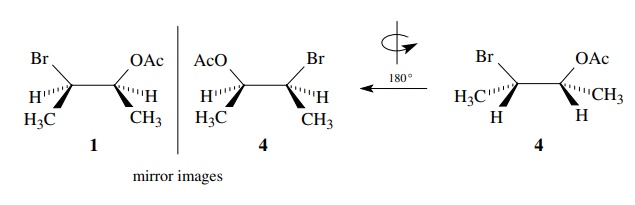
but nonsuperimposable and therefore are enantiomers.

A similar analysis reveals that 2 and 3 are also enantiomers. Comparison of any other pairs of stereoisomers, 1 and 2, for example, shows that they are not mirror images: The C-2 of 1 is R and C-2 of 2 is S but C-3 of both 1 and 2 is S. Isomers 1 and 2 are also not superimposable. So 1 and 2 are a second type of stereoisomer and are nonsuperimposable, non-mirror images called diastereomers. Diastereomers have the same molecular formula and sequence of bonded elements but different spatial arrangements and are nonsuperimposable, non-mirror images.

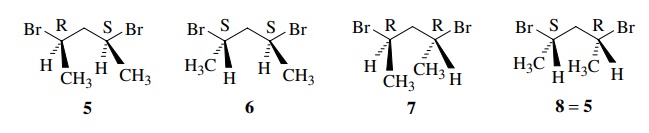
A third type of stereoisomer occurs when a molecule with several stere-ogenic centers contains an internal plane of symmetry. This usually happens when two of the stereogenic centers are attached to the same four different valences. For example, 2,4-dibromopentane has two stereogenic centers and thus four stereoisomers, 5–8. It is easily seen that 6 and 7 are enantiomers, 5 and 6 are diastereomers, and so on.

However, 5 and 8 are identical. Although there are two chiral centers in 5 (and 8), the molecule itself is achiral because it contains an internal mirror plane. Thus it has a plane of symmetry. Structure 8 is superimposable on 5 by a 180^◦ rotation and thus is the same compound. This molecule is called a meso isomer, a compound which contains chiral centers but itself has a plane of symmetry. Even though 2,4-dibromopentane has two stereogenic centers, there are really only three stereoisomers, a pair of enantiomers and a meso compound which is diastereomeric with the enantiomeric pair.

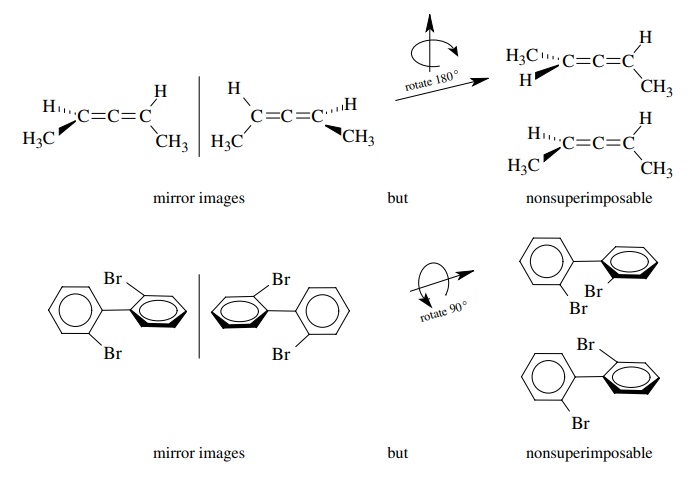
It is clear from the above examples that the presence of chiral centers in molecules leads to stereoisomers. There is another type of molecule which itself is chiral but has no chiral center. The molecular chirality arises from the presence of a screw axis in the molecule. Allenes and biphenyls are common examples of such compounds, and because they are chiral, they exist as enantiomers.

We have seen that when more than one stereocenter is present in a molecule, both enantiomers and diastereomers are possible. Distinguishing between enan-tiomers requires the relative configurations of each stereogenic center to be specified. However, to distinguish diastereomers, only the relative spatial ori-entation of groups needs to be specified. For example, aldotetroses have two stereocenters and the four stereoisomers are shown below:

The enantiomeric relationship between D-threose and L-threose is specified by the 2S,3R and 2R,3S configurations (each stereocenter is the mirror image of the other). Moreover the enantiomeric relationship between D-erythrose and L-erythrose is clear from the 2R,3R and 2S,3S configurations. However, threose and erythrose are diastereomers. The different spatial orientation of the –OH groups extending from the chain in the Fisher projections makes the diastereomeric relationship obvious without the need for specifying the configuration; that is, they are clearly nonsuperimposable and non-mirror images.

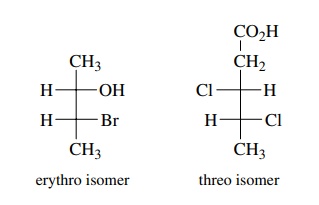
By extension, other diastereomeric pairs of molecules which contain two adja-cent stereogenic centers can be designated as threo or erythro depending on whether substituents extend to opposite (threo) or the same (erythro) sides of the Fisher projection of the molecule. For example,

The threo and erythro designation denotes a diastereomeric relationship of the isomers. Each threo and erythro isomer will also have enantiomers which will also have a threo – erythro diastereomeric relationship to each other.

More recently a new method for designating the stereochemical relationship of diastereomers has been developed. In this method the carbon backbone is extended in the plane of the paper, blackboard, or computer screen in the hor-izontal direction. Groups will extend from this backbone either in front of the plane or behind it and are designated by bold or dashed bonds, respectively. If two substituents extend in the same direction, their spatial relationship is designated syn; if they extend in opposite directions, their spatial relationship is designated anti.

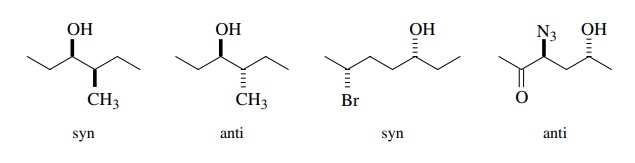
Molecules which are syn – anti isomers of each other are diastereomers, and there will be two syn enantiomers and two anti enantiomers. The syn – anti designation is not restricted to substituents on vicinal carbon atoms as is the threo – erythro designation and is thus more versatile.